The "shell method" is a powerful technique in calculus used to find the volume of a solid of revolution. By revolving a region around an axis, this method simplifies complex volume calculations, making it an essential tool for students, mathematicians, and engineers alike. The "shell method" is particularly valuable when dealing with cylindrical shells, offering an alternative to the disk or washer methods. It provides a unique approach to solving problems that would otherwise require intricate calculations, saving both time and effort.
Unlike other methods, the "shell method" focuses on using the formula for the lateral surface area of cylindrical shells to determine volume. This technique is especially effective for regions that are more conveniently integrated along one axis rather than the other. Whether you're a student preparing for exams or a professional working in a field that requires precise volume measurements, mastering the "shell method" can significantly enhance your problem-solving skills.
In this guide, we'll take a deep dive into the fundamentals of the "shell method," explore its applications, and answer some of the most common questions about this technique. By the end of this article, you'll have a thorough understanding of what the "shell method" is, how to apply it, and why it's such an indispensable tool in the world of calculus.
Read also:Norwegian Prayer A Timeless Spiritual Tradition And Its Profound Significance
Table of Contents
- What Is the Shell Method?
- Why Is the Shell Method Important?
- How Does the Shell Method Work?
- When Should You Use the Shell Method?
- Step-by-Step Guide to Using the Shell Method
- Examples of Shell Method Calculations
- Common Mistakes When Using the Shell Method
- Advantages of the Shell Method
- Disadvantages of the Shell Method
- How Does the Shell Method Compare to the Disk Method?
- Applications of the Shell Method
- Can the Shell Method Be Used in Other Fields?
- Real-World Examples of the Shell Method
- Tips and Tricks for Mastering the Shell Method
- Frequently Asked Questions About the Shell Method
What Is the Shell Method?
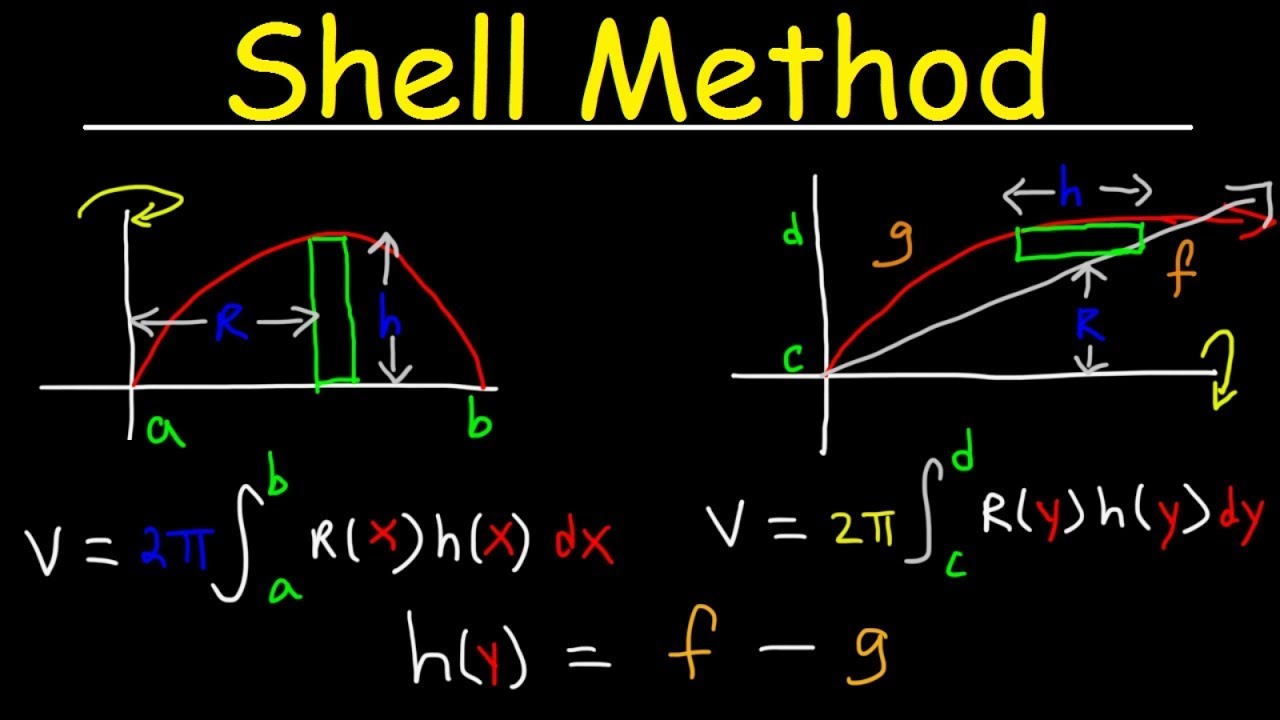
The "shell method" is a calculus technique used to calculate the volume of a solid of revolution. It works by dividing the solid into cylindrical shells and summing their volumes using integration. This method is particularly useful for solids that are easier to analyze when rotated around a specific axis.
Why Is the Shell Method Important?
The "shell method" is important because it provides an alternative approach to volume calculation, especially when the disk or washer methods are not convenient. It simplifies the process, making it easier to solve complex problems in calculus and engineering.
How Does the Shell Method Work?
The "shell method" works by integrating the volume of cylindrical shells. The formula for the volume of a shell is:
- V = 2π ∫ [radius × height] dx (or dy)
The radius and height of each shell depend on the position and dimensions of the region being revolved.
When Should You Use the Shell Method?
You should use the "shell method" when the region being revolved is easier to integrate along one axis than the other. It’s also ideal when the boundaries of the region are more naturally expressed in terms of one variable.
Step-by-Step Guide to Using the Shell Method
Follow these steps to apply the "shell method" effectively:
Read also:All You Need To Know About The Unique Beauty Of Dapple Piebald
- Identify the axis of revolution.
- Determine the boundaries of the region to be revolved.
- Set up the integral using the formula for the volume of a shell.
- Evaluate the integral to find the volume.
Examples of Shell Method Calculations
Here are some examples to illustrate the "shell method":
- Example 1: Calculating the volume of a solid revolved around the y-axis.
- Example 2: Using the "shell method" for a region bounded by a curve and a line.
Common Mistakes When Using the Shell Method
Avoid these common mistakes when using the "shell method":
- Incorrectly identifying the radius or height of the shell.
- Setting up the wrong limits of integration.
- Forgetting to multiply by 2π in the formula.
Advantages of the Shell Method
The "shell method" offers several advantages:
- Simplifies calculations for certain solids of revolution.
- Provides a flexible alternative to the disk and washer methods.
- Works well for regions described in terms of one variable.
Disadvantages of the Shell Method
Despite its benefits, the "shell method" has some limitations:
- Can be confusing for beginners.
- Requires understanding of integration techniques.
How Does the Shell Method Compare to the Disk Method?
The "shell method" and disk method are both used to calculate volumes, but they differ in their approach. The shell method is more suitable for problems where integration along a specific axis is easier, while the disk method works better for perpendicular integration.
Applications of the Shell Method
The "shell method" is widely used in fields such as engineering, physics, and architecture. It is particularly valuable for designing structures and analyzing fluid dynamics.
Can the Shell Method Be Used in Other Fields?
Yes, the "shell method" has applications beyond calculus. It is used in computer graphics, manufacturing, and even medical imaging to calculate volumes and shapes.
Real-World Examples of the Shell Method
Here are some real-world scenarios where the "shell method" is applied:
- Designing cylindrical storage tanks.
- Analyzing the volume of irregularly shaped objects.
Tips and Tricks for Mastering the Shell Method
To master the "shell method," keep these tips in mind:
- Practice with a variety of problems.
- Visualize the region and shells before setting up the integral.
- Review integration techniques to ensure accuracy.
Frequently Asked Questions About the Shell Method
Here are some commonly asked questions about the "shell method":
- What is the main advantage of the "shell method" over the disk method?
- Can the "shell method" be used for solids with complex boundaries?
- How do you determine the limits of integration for the "shell method"?